|
 "The
great part of the miseries of mankind are brought upon them by false estimates they have made of the value of things." "The
great part of the miseries of mankind are brought upon them by false estimates they have made of the value of things."
-- Benjamin Franklin
One of the great myths of poker is the assertion "Omaha hands run close together in value". Demonstrating the absurdity of this
statement is easy, but a more fundamental problem for the people who parrot this sentiment is the lack of a practical understanding of both
the concepts of value and equity. (The examples are High-Low, but the concepts involved apply to High-Only just as well.)
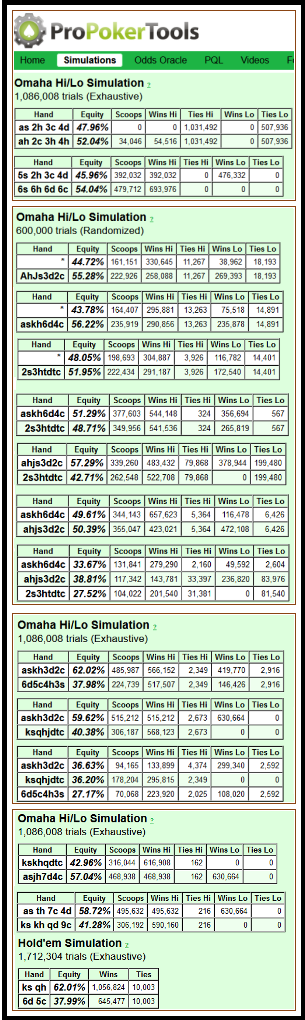
Let's start with the several Omaha High-Low examples that are displayed at right. The first example compares A234offsuit versus
Ax2x3♠4♠. Obviously when played against each other, these two hands will normally split the pot, but deciding these two hands run
close together in value based on the 47.96%/52.04% in "equity" is idiotic. Ax2x3♠4♠ is an infinitely better hand head-up.
The second example should make an even more important point. When comparing 5432offsuit versus 6666, we find the 6666 is actually a
54.04% favorite. But this faux-equity offers us zero information about real value. In a hand with betting, 5432 will destroy 6666, largely
because 6666 will never call any bet, ever. The only value that 6666 can extract is when the player with 5432 chooses never to bet.
Real value, actual equity, can only be considered when betting is included in the calculations.
Likewise, on a 765 flop, A234offsuit and 89TJoffsuit would show as exactly tied in simulated equity, but now suppose a flush card that
pairs the board comes on the turn or river... and suppose this is a
PLO8 hand. The idea that these hands had the same
equity on this flop is idiotic. Hands like 89TJ often can not stand the heat of actual betting, and even if they do, the longterm
$-value of the hands will be drastically different.
True value is found in wagering. In both limit and pot limit Omaha, hands that have clear nut-holdings (like nut low or nut
flush hands) that can stand the heat of wagering, especially heavy wagering, have a great deal more value and are able to extract
their true equity far better than hands that are more vulnerable or more speculative.
Truly valuable Omaha hands are ones that can make it to the showdown to extract their share of the pot, while also not playing with a
negative expectation to get there (like 89TJ would have to when calling multiple bets on a 7657A board).
Many hands that simulate as 45/55 underdogs actually perform far worse than that (or even 54/46 favorites like 6666 versus 5432.) The
miserable hand of 2222 will have a negative equity on any flop if there is betting, no matter what equity percentage a simulation will
show. That's an extreme example, but there are many hands, especially when played by a novice player, that will have an actual
negative value even in the big blind in an unraised pot.
Some hands have to play highly negative expectation post-flop to extract their simulated equity on the flop. Look at the AJ32 vs.
TT32 vs. AK64 confrontations. The first three simulations show that against a random hand AK64 > AJ32 > TT32, with TT32 barely better
than a random hand. The next three simulations reveal AK64 is a minor favorite over TT32, AJ32 is a micro-favorite over AK64, and AJ32
is a major favorite over TT32. Then finally, three ways AK64 is just a bit better than average at 33.67%, while AJ32 is dominating
with 38.81% in comparison to the TT32 at 27.52%.
TT32 is weaker in the simulations than the other two hands, but this is again without taking betting into consideration.
Like the "pretty" sucker hands that are overvalued by
weaker Texas Hold'em players, Omaha High-Low hands like TT32 look enticing to players who fall for their superficial appeal. To earn
it's full simulated equity share, TT32 will have to call bet-bet-bet on flops like 863Q9 to split against AJ32 or AK64. In contrast,
the latter two hands will face far fewer pathetic call situations to the river. A key reason
playing "five-card hands" is the Secret of Omaha, they
offer far more flexibility to get their full value sensibly than four card hands do. (Hands like AK64 are "five card hands" because
they have two high cards, Ace and King, and three low cards, Ace and six and four.)
Thinking of Omaha hands as six two-card Hold'em hands isn't a great way to approach the game, but it is helpful in understanding why
"five-card hands" like AJ32 are greatly superior to four-card hands like TT32 in being able to extract their full simulated value
(and more). The six high-low Hold'em hands in AJ32 are AJ, A2, A3, 23 and the weak J3 and J2. In contrast, TT32 can only make TT and 32,
plus the weak T3 and T2. TT32 has a lot less ways to solidly connect two cards to a flop. Having two redundant versions of T3 and T2
in your hand is not helpful! (Having TT32 double-suited helps as now at least the T2 and T3 have some minimal value.)
The more ways you can hit the flop, the more ways you can continue playing with pot odds and a positive expectation, which means you have more
paths to get to not just your equity share, but also the equity of players whose less flexible hands don't allow them to make post-flop EV+ bets.
The second-to-last group of simulations contrast AK32 with KQJT and 6543. AK32 is about a 60% favorite head-up against the other two
hands. The interesting thing though is three-way, AK32 and KQJT perform about the same, while (not shown) 6543 is about a 10% favorite
head-up over KQJT. What this demonstrates is the prime quality five-card hand of AK32 can basically fight any battle, while the four card
hands of KQJT and 6543 can have the best of it sometimes, under the right circumstances, even in different ways against each other. When playing
short-handed, playing hands that are normally garbage can make sense, but in full-table situations, they both suffer from inflexibility...
though KQJT can be nicely EV+ in a limited number of situations, whereas 6543 will hardly ever get EV+ situations at a full table of decent players.
Finally, the last group of simulated High-Low hands are KKQT or KKQ9 versus one of the weakest five-card hands, AT74. The concepts
above all come in play here. While KKQT is a fine hand in a multiway pot, it is dreadful head-up against a five-card hand as weak as
AT74. These Broadway-card hands are dreadful both because their basic simulation numbers are only in the 41-43% range, but because
they have an even harder time to stand the heat of betting in an EV+ way than TT32 does.
How do you like calling bets with KKQT on an 873 flop? If you want to get your simulated value against AT74, you have to do just
that. In contrast, if you play the hand, and sensibly fold the flop, you are also abandoning whatever your pot equity is -- no matter
what the simulation told you before the flop. These limited hands lead to a lot of "damned if you do, damned if you don't"
situations. Don't paint yourself in a corner. Play hands with escape hatches.
I included the Hold'em simulation at the end to show KKQ9 simulates only 3.29% better against AT74 than 65o does against KQo... but given
the bet-ability factors above, KKQ9 probably plays significantly worse in Limit against AT74 than 65o does against KQo. Not too many people
assert "65o and KQo run close together in value", so there is no reason to say such nonsense about Omaha hands.
The bottom line is you have to get to the showdown to realize preflop simulated equity.
To get real value, play hands that are flexible and can stand the heat of betting. Play hands that are flexible, have multiple ways to win
and can continue in an EV+ way on the vast majority of flopped boards. Play hands where you can make reasonable bets that force players with
limited hands to choose between abandoning their equity or continuing with EV- hands.
See also Good Variance vs. Bad Risk,
Omaha Myths and
The Secret of Omaha.
Simulations from ProPokerTools |

